The most common questions we receive are about how a spacecraft stays in orbit. More specifically, the physics and mathematics involved.
In this first part, we will delve deep into the orbiting phenomenon. In the second one, instead, we will discuss other hot topics linked to how spacecrafts orbit.
So let’s get to the first question: How exactly do satellites stay in orbit?
Qualitative Description of the Phenomenon
Satellites are able to stay in orbit thanks to their velocity. However, let’s take this one step further.
In the XVII century, Sir Isaac Newton was able to explain how satellites remain in Earth’s orbit starting from the concept of projectile motion. For example, if you throw a stone from a mountaintop, it will fall on the ground at some set distance. If you then throw that same stone from the same height but with greater speed, it will now travel further than it did in the first throw. Now, let’s expand this example to include a much larger scale, such as Earth. As our beloved planet is not flat, a projectile that has enough speed will continue to “fall” without ever intersecting the ground.
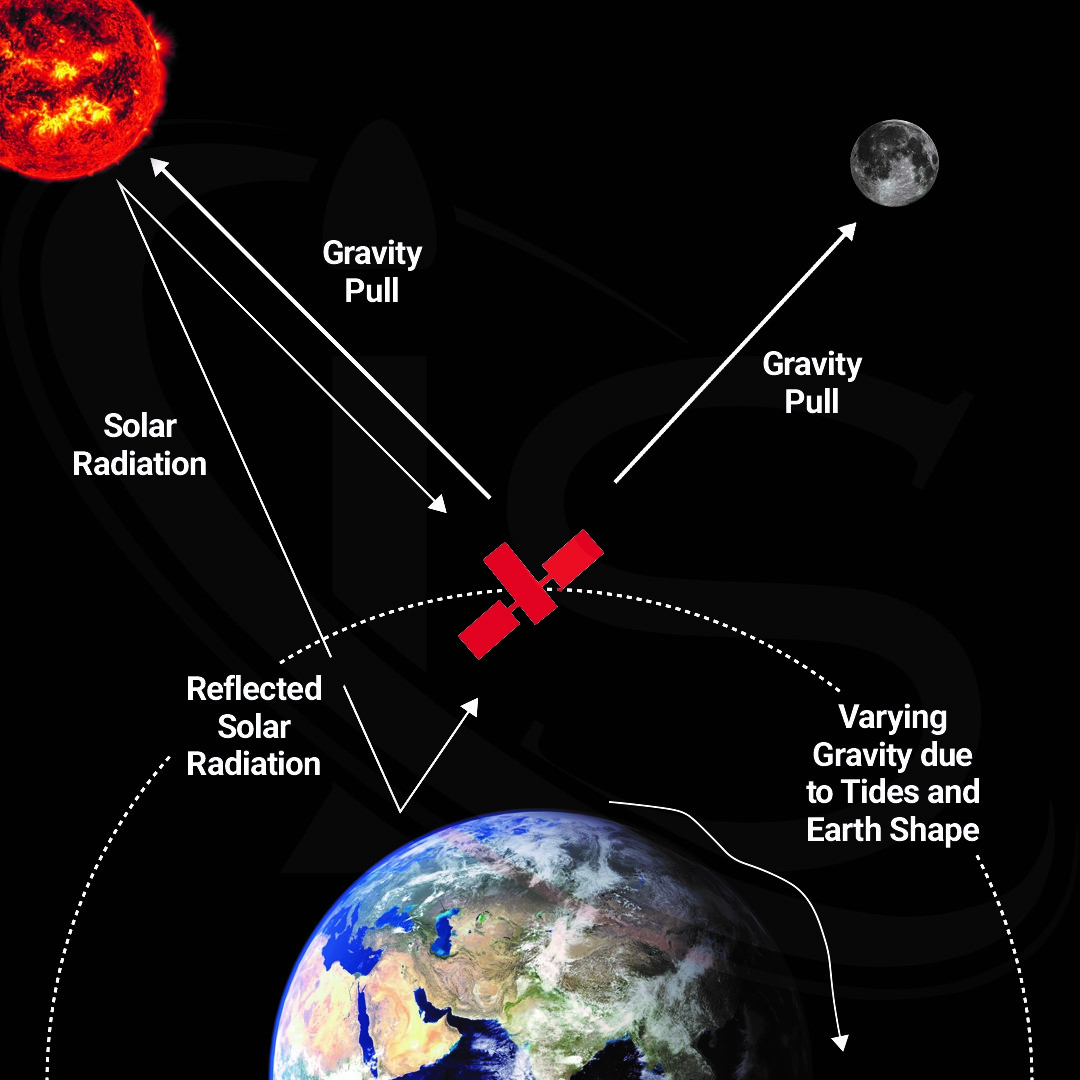
So, a satellite that fulfills these conditions will end up traveling around the Earth, meaning that it will start orbiting our planet. As satellites are placed outside the Earth’s atmosphere where there is no resistance due to air — also known as drag — they are able to stay orbiting our planet for many years. Furthermore, due to Newton’s first law, an object that moves at a constant speed will stay at said constant speed unless acted upon by some outside force. And that is how satellites are able to maintain a constant orbit around the Earth. Of course, this is an oversimplification, as we are not accounting for every force that’s acting on them — such as solar radiation pressure, and the gravitational pulls of the Sun and Moon. However, we have many capsules in store that will delve deeper into these topics, so stay tuned for those!
Still, the following question arises:
How fast do these satellites have to go?
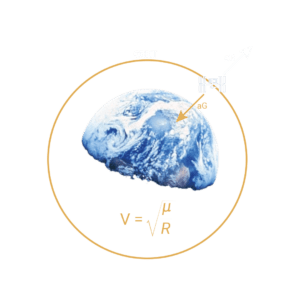
To answer this, let’s take the case of a circular orbit. To obtain the velocity needed for the satellite to remain in orbit, we need to equate the gravitational force with the pseudo outward “force”, commonly known as the centrifugal force. The equations for these two quantities are, respectively:
![Rendered by QuickLaTeX.com \[ \mu\frac{m}{R^2} = \frac{mV^2}{R} \ \longrightarrow \ V = \sqrt{\frac{\mu}{R}},\]](https://impulso.space/wp-content/ql-cache/quicklatex.com-994592706e76116f3959dc0228462f01_l3.png)
where ![]() is the standard gravitational parameter.
is the standard gravitational parameter.
Therefore, the only variable influencing the velocity of a circular orbit is the radius from the center of the Earth to the orbit itself.
For a practical example, we can look at the International Space Station or ISS, which, in order to stay at an orbit of 400 km above the Earth’s surface, needs the following velocity of V = 7.8 km/s = 28,000 km/h or 17,500 mi/h. That is because the R in this problem is given by Earth’s radius plus the orbiting distance. Meaning, R = 6371 +400 = 6771 km.
To better understand the change in velocity between two different orbits, it could be useful to also see what is the required velocity for a satellite in a GEO orbit (for more info about this orbit check the Altitude Classification). We have to repeat the previous calculation for an orbit, but with an altitude of 35,786 km. This corresponds to a radius of R = (6,371 + 35,786) km = 42,157 km. The resulting velocity is then V = 3.075 km/s =11,070 km/h.
So, between an orbit of 400 km and one of 35,786 km, the delta between the two velocities is ΔV = (28,000 – 11,000) km/h = 17,000 km/h. More than twice as slow!
And now it is time to proceed to the most common questions linked to this topic…
What keeps a satellite up in its orbit?
In the previous paragraph, we just touched on this point by explaining the math behind the velocity of a spacecraft. But this topic is very interesting and meaningful when it comes to a spacecraft’s orbit.
As we saw before, the satellite needs to have a centrifugal “force” that equalizes the gravitational pull. This centrifugal “force” is a pseudo force that appears to act on all objects in a rotating frame of reference. And, in this particular case, the speed of the spacecraft generates is what generates it. If the spacecraft is sent into orbit too slowly, the centrifugal force will not balance the gravitational force, causing the spacecraft to fall back down to Earth. So the answer is: “Nothing keeps the satellite into orbit. It is simply a matter of setting the speed that generates the appropriate centrifugal force to balance out the gravitational pull”.
Do satellites eventually fall out of orbit?
This question is really interesting and, in fact, “yes, satellites could fall out of their orbit”. This phenomenon can be intentional or “casual”.
In the first case, the fall of a satellite can be imposed from the satellite operator; this can be due to damage on the satellite or to the end of its lifespan. To impose the fall of a satellite you need to have the possibility to change the speed of it, e.g. with a propulsion system.
In the second case, the satellite can have some trouble reaching orbit, something that happened some months ago with the launch of a Starlink batch which had to reenter the atmosphere due to a solar storm. The satellites reentering the atmosphere were destroyed.
However, this is not the only case for de-orbiting. Another very important, already mentioned in this article one is the ISS. The ISS has such a great mass (more than 400,000 kg!) and volume that atmospheric drag plays a bigger role here. In fact, the ISS’s orbit decays 2 km every month. After all, this year the ISS should have already crashed back to Earth. However, thank to its own propulsion, it is able to counter this decay.
That is it for this Technical Capsule on “How do satellites stay in orbit?”. If you are interested in other aspects of satellites please check out the Satellite tag on our Blog page.